Video

Quiz

Hier geht's zum Video „Binomische Formeln“

Hier geht's zum Video „Pascalsches Dreieck“

Binomische Formel hoch 3

Wichtige Inhalte in diesem Video

Binomische Formel mit Exponent 3 einfach erklärt

Binomische Formel hoch 3

Binomische Formeln hoch 4

Binomische Formeln hoch 5

Mit den binomischen Formeln kannst du leicht Klammern mit einem hoch 2 ausmultiplizieren, aber wie funktioniert das bei x hoch 3, x hoch 4 oder sogar x hoch 5? Das zeigen wir dir hier. Schaue dir auch unser passendes Video dazu an

Inhaltsübersicht

Binomische Formel mit Exponent 3 einfach erklärt

im Videozur Stelle im Video springen

Du kennst wahrscheinlich schon die 1. binomische Formel (a+b)²=a²+2ab+b² und die 2. binomische Formel (a-b)²=a²-2ab+b². Sie sind eine praktische Hilfe beim Ausrechnen und Umformen von Gleichungen, aber sie funktionieren nur bei x hoch 2. Was machst du, wenn der Exponent deines Binoms größer als 2 ist?

Zum Glück gibt es auch binomische Formeln hoch 3.

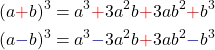
Binomische Formeln

$\begin{align*} (a\textcolor{red}{+}b)^3 &= a^3 \textcolor{red}{+} 3a^2b \textcolor{red}{+} 3ab^2 \textcolor{red}{+} b^3 \\ (a \textcolor{blue}{-}b)^3 &= a^3 \textcolor{blue}{-} 3a^2b\textcolor{red}{+}3ab^2\textcolor{blue}{-}b^3 \end{align*}$

Binomische Formel hoch 3

im Videozur Stelle im Video springen

Schaue dir zum Beispiel die Formel (a+b)³. Um binomische Terme mit dem Exponent 3 zu vereinfachen, rechnest du zuerst die Potenz aus. Dafür schreibst du deinen Term als Produkt von (a+b) und einer Klammer hoch 2 der ersten binomischen Formel (a+b)².

$(a+b)^3 = (a+b)\cdot (a+b)^2$

Die erste binomische Formel kennst du schon und kannst sie auflösen. Du ersetzt deshalb die Klammer hoch 2 durch (a+b)² = (a²+2ab+b²):

$(a+b)^3 = (a+b)\cdot (a^2+2ab+b^2)$

Als nächstes multiplizierst du die übrigen zwei Klammern aus. Du rechnest also (a+b) mal jeden Term in der zweiten Klammer (a², 2ab und b²). Danach musst du noch einmal ausmultiplizieren, indem du die (a+b)-Klammern auflöst. Du bekommst dann zunächst einen langen und komplizierten Ausdruck.

$\begin{align*} (a+b)^3 &= (a+b)\cdot (a^2+2ab+b^2) \\ (a+b)^3 &= (a+b)\cdot a^2 + (a+b)\cdot 2ab + (a+b)\cdot b^2 \\ (a+b)^3 &= a^3+\textcolor{blue}{a^2b} +\textcolor{blue}{2a^2b}+\textcolor{red}{2ab^2} + \textcolor{red}{ab^2}+b^3 \end{align*}$

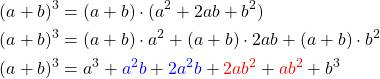
Du kannst ihn aber noch vereinfachen, indem du die farbigen Terme zusammenfasst. Dann bekommst du die binomische Formel mit dem Exponent 3 heraus:

$(a+b)^3 = a^3 + \textcolor{blue}{3a^2b} + \textcolor{red}{3ab^2} + b^3$

Schauen wir uns noch ein paar Beispiele dazu an. Was ist das Ergebnis von (x+1)³? Das kannst Du mit der binomischen Formel hoch 3 ganz einfach lösen!

$(x+1)^3 = x^3 + 3x^2 + 3x + 1$

Natürlich kannst du auch die 2. binomische Formel hoch 3 rechnen. Was ist zum Beispiel (x-1)³?

$(x-1)^3 = x^3 -3x +3x -1$

Mathe Binomische Formeln hoch 3

$\begin{align*} (a\textcolor{red}{+}b)^3 &= a^3 \textcolor{red}{+} 3a^2b \textcolor{red}{+} 3ab^2 \textcolor{red}{+} b^3 \\ (a \textcolor{blue}{-}b)^3 &= a^3 \textcolor{blue}{-} 3a^2b\textcolor{red}{+}3ab^2\textcolor{blue}{-}b^3 \end{align*}$

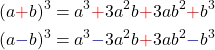
Binomische Formeln hoch 4

im Videozur Stelle im Video springen

Auf die gleiche Weise kannst du deine Aufgaben ausrechnen, wenn du eine Binom. Formel hoch 4 auflösen magst. Du kannst hoch 4 auch als deine binomische Formel hoch 3 mal (a+b) schreiben.

$(a+b)^4 = (a+b)\cdot(a+b)^3$

Das Ergebnis von (a+b)³ kennst du schon. Das kannst du jetzt wie vorher mit der Klammer multiplizieren.

$\begin{align*} (a+b)^4 &= (a+b)\cdot (a^3 + 3a^3b +3ab^2 + b^3) \\ (a+b)^4 &= a^4 + 4a^3b + 6a^2b^2 + 4ab^3 + b^3 \end{align*}$

Mathe Binomische Formeln hoch 4

$\begin{align*} (a\textcolor{red}{+}b)^4 &= a^4 \textcolor{red}{+} 4a^3b \textcolor{red}{+} 6a^2b^2 \textcolor{red}{+} 4 ab^3 \textcolor{red}{+} b^4\\ (a\textcolor{blue}{-}b)^4 &= a^4\textcolor{blue}{-} 4a^3b \textcolor{red}{+} 6a^2b^2 \textcolor{blue}{-} 4 ab^3 \textcolor{red}{+} b^4 \end{align*}$

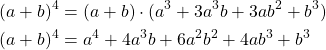
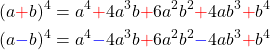
Schaue dir das am besten an einem Beispiel an: Was ist (x+2)⁴ und (x-2)⁴?

$\begin{align*} (x+2)^4 &= x^4 + 8x^3 + 24x^2 + 32x + 16 \\ (x-2)^4 &= x^4 - 8x^3 + 24x^2 -32x + 16 \end{align*}$

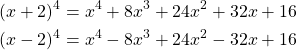
Binomische Formeln hoch 5

im Videozur Stelle im Video springen

Eine Binom. Formel hoch 5 wird dir wahrscheinlich sehr selten begegnen, aber für alle Fälle haben wir dir auch dafür die richtige Formel ausgerechnet. Du kannst sie wie die binomische Formel hoch 3 und hoch 4 auch per Hand ausrechnen.

Mathe Binomische Formeln hoch 5

$\begin{align*} (a\textcolor{red}{+}b)^5 &= a^5 \textcolor{red}{+} 5a^4b \textcolor{red}{+} 10a^3b^2 \textcolor{red}{+} 10 a^2b^3 \textcolor{red}{+} 5ab^4 \textcolor{red}{+} b^5\\ (a\textcolor{blue}{-}b)^5 &= a^5 \textcolor{blue}{-} 5a^4b \textcolor{red}{+} 10a^3b^2\textcolor{blue}{-} 10 a^2b^3 \textcolor{red}{+} 5ab^4 \textcolor{blue}{-} b^5\\ \end{align*}$

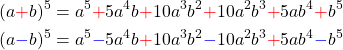
Auch hierfür haben wir dir ein Beispiel ausgerechnet. Was ist das Ergebnis, wenn du (x+2)⁵ mit der binomischen Formel hoch 5 umformst?

$(x+2)^2 = x^5 + 10x^4 + 40x^3 + 80x^2 + 80x + 32$

Quiz zum Thema Binomische Formel hoch 3

Pascalsches Dreieck

Wenn du noch höhere Exponenten ausrechnen willst, hilft dir das Pascalsche Dreieck weiter. Daran kannst du sofort erkennen, wie deine binomischen Formeln aussehen. Schaue dir gleich unser Video dazu an!

Zum Video: Pascalsches Dreieck

zur Videoseite: Binomische Formel hoch 3

Beliebte Inhalte aus dem Bereich Mathe Grundlagen

Binomische Formeln

2. binomische Formel

3. binomische Formel

Binomische Formeln Aufgaben

Pascalsches Dreieck

Binomische Formel hoch 3

Hallo, leider nutzt du einen AdBlocker.

Auf Studyflix bieten wir dir kostenlos hochwertige Bildung an. Dies können wir nur durch die Unterstützung unserer Werbepartner tun.

Schalte bitte deinen Adblocker für Studyflix aus oder füge uns zu deinen Ausnahmen hinzu. Das tut dir nicht weh und hilft uns weiter.

Danke!
Dein Studyflix-Team

Wenn du nicht weißt, wie du deinen Adblocker deaktivierst oder Studyflix zu den Ausnahmen hinzufügst, findest du hier eine kurze Anleitung. Bitte .