Vektorrechnung
Du möchtest die Vektorrechnung in ihren Grundlagen verstehen und einige Aufgaben dazu rechnen? Dann übe am besten mit diesem Artikel und dem entsprechenden Video einige Rechnungen mit Vektoren.
Inhaltsübersicht
Vektorrechnung einfach erklärt
Erinnere dich kurz, was Vektoren sind:
Ein Vektor ist eine Menge von Pfeilen, die
- zueinander parallel sind,
- in dieselbe Richtung zeigen (dieselbe Orientierung besitzen) und
- gleich lang sind.
Die Länge eines solchen Pfeils nennst du den Betrag
| |des Vektors
|des Vektors  . Du berechnest ihn so:
. Du berechnest ihn so:
![Rendered by QuickLaTeX.com \[|\vec{x}| = \left| \begin{pmatrix} \textcolor{red}{1} \\ \textcolor{blue}{2} \\ \textcolor{olive}{3} \end{pmatrix} \right| = \sqrt{\textcolor{red}{1}^2 + \textcolor{blue}{2}^2 + \textcolor{olive}{3}^2} = \sqrt{1 + 4 + 9} = \sqrt{14} \approx 3.74 \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-24a7c6f33644268e1c506112dac9225b_l3.png)
Du kannst Vektoren addieren.
![Rendered by QuickLaTeX.com \[\textcolor{red}{\begin{pmatrix} 1 \\ 3 \\ 6 \end{pmatrix}} + \textcolor{blue}{\begin{pmatrix} 2 \\ 4 \\ 5 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{1} + \textcolor{blue}{2} \\ \textcolor{red}{3} + \textcolor{blue}{4} \\ \textcolor{red}{6} + \textcolor{blue}{5} \end{pmatrix} = \begin{pmatrix} 3 \\ 7 \\ 11 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-364bc8d571a8fe712669caf2ace8615b_l3.png)
Du kannst sie mit einer Zahl multiplizieren.
![Rendered by QuickLaTeX.com \[\textcolor{red}{4} \cdot \textcolor{blue}{\begin{pmatrix} 2 \\ 0 \\ 5 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{4} \cdot \textcolor{blue}{2} \\ \textcolor{red}{4} \cdot \textcolor{blue}{0} \\ \textcolor{red}{4} \cdot \textcolor{blue}{5} \end{pmatrix} = \begin{pmatrix} 8 \\ 0 \\ 20 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-e16b726671052ce88de4f69b6162d52d_l3.png)
Aber du kannst auch das Skalarprodukt
![Rendered by QuickLaTeX.com \[ \textcolor{red}{\begin{pmatrix} 1 \\ 8 \\ 7 \end{pmatrix}} \circ \textcolor{blue}{\begin{pmatrix} 2 \\ 5 \\ 6 \end{pmatrix}} = \textcolor{red}{1} \cdot \textcolor{blue}{2} + \textcolor{red}{8} \cdot \textcolor{blue}{5} + \textcolor{red}{7} \cdot \textcolor{blue}{6} = 2 + 40 + 42 = 84 \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-60b794bece04caa60861377e97ed75aa_l3.png)
oder das Kreuzprodukt berechnen.
![Rendered by QuickLaTeX.com \[\textcolor{red}{\begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix}} \times \textcolor{blue}{\begin{pmatrix} 4 \\ 5 \\ 6 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{2} \cdot \textcolor{blue}{6} - \textcolor{red}{3} \cdot \textcolor{blue}{5} \\ \textcolor{red}{3} \cdot \textcolor{blue}{4} - \textcolor{red}{1} \cdot \textcolor{blue}{6} \\ \textcolor{red}{1} \cdot \textcolor{blue}{5} - \textcolor{red}{2} \cdot \textcolor{blue}{4} \end{pmatrix} = \begin{pmatrix} 12 - 15 \\ 12 - 6 \\ 5 - 8 \end{pmatrix} = \begin{pmatrix} -3 \\ 6 \\ -3 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-0ae36bbf0b6e0dd8a2a24954e444468a_l3.png)
Vektorrechnung Addition
Bei der Vektoraddition addierst du zeilenweise die Einträge der Vektoren miteinander.
![Rendered by QuickLaTeX.com \[\textcolor{red}{\begin{pmatrix} 1 \\ 3 \\ 6 \end{pmatrix}} + \textcolor{blue}{\begin{pmatrix} 2 \\ 4 \\ 5 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{1} + \textcolor{blue}{2} \\ \textcolor{red}{3} + \textcolor{blue}{4} \\ \textcolor{red}{6} + \textcolor{blue}{5} \end{pmatrix} = \begin{pmatrix} 3 \\ 7 \\ 11 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-20212655257bdc0f57261f723427a4a2_l3.png)
Geometrisch kannst du dir die Addition in der Vektorenrechnung so vorstellen, dass du den Anfang eines Vektors an das Ende (die Spitze) des anderen Vektors klebst.
Das Ergebnis von  ist dann der direkte Weg zwischen dem Schaft von
ist dann der direkte Weg zwischen dem Schaft von  und der Spitze von
und der Spitze von  .
.
Du kannst mit der Subtraktion von zwei Punkten A und B den Vektor ausrechnen, der A und B verbindet. Das ist der Verbindungsvektor  .
.
![Rendered by QuickLaTeX.com \[\overrightarrow{AB} = \overrightarrow{B} - \overrightarrow{A}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-e55342a83df752fdb72dc50de0de620e_l3.png)
Also ist der Verbindungsvektor zwischen A = (2 | 8 | 5) und B = (1 | 4 | 3):
![Rendered by QuickLaTeX.com \[\overrightarrow{AB} = \overrightarrow{B} - \overrightarrow{A} \begin{pmatrix} 1 \\ 4 \\ 3 \end{pmatrix} - \begin{pmatrix} 2 \\ 8 \\ 5 \end{pmatrix} = \begin{pmatrix} 1 - 2 \\ 4 - 8 \\ 3 - 5 \end{pmatrix} = \begin{pmatrix} -1 \\ -4 \\ -2 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-a276d3f016be42b4f2d175368575ea48_l3.png)
Der Abstand zwischen A und B ist die Länge des Verbindungsvektors  .
.
In unserem Beispiel ist der Abstand zwischen A und B also:
![Rendered by QuickLaTeX.com \[|\overrightarrow{AB}| = \left| \begin{pmatrix} -1 \\ 4 \\ 2 \end{pmatrix} \right| = \sqrt{(-1)^2 + (-4)^2 + (-2)^2} = \sqrt{1 + 16 + 4} = \sqrt{21} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-7933560b69ae4bde7c40009c427b23ed_l3.png)
Vektorrechnung Addition Aufgaben:
- Berechne:
![Rendered by QuickLaTeX.com \[\begin{pmatrix}2 \\5 \\7\end{pmatrix}+\begin{pmatrix}1 \\6 \\3\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-3d4c8f4059e7040a82216212d34d2026_l3.png)
- Berechne den Abstand der beiden Punkte A(4|2|0) und B(0|8|1).
- Du bedienst eine ferngesteuerte Drohne. Du lässt sie zuerst 30m nach oben fliegen. Dann 10m nach rechts. 2m nach unten. 7m nach links. 8m nach vorne. Und wieder 2m nach oben. Wie weit ist die Drohne von ihrem Startpunkt entfernt? (fortgeschritten)
Vektorrechnung Addition Lösungen:
- Komponentenweise Addieren ergibt:
![Rendered by QuickLaTeX.com \[\begin{pmatrix}2 \\5 \\7\end{pmatrix}+\begin{pmatrix}1 \\6 \\3\end{pmatrix}=\begin{pmatrix}2 + 1\\5 + 6\\7 + 3\end{pmatrix}=\begin{pmatrix}3 \\11 \\10\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-e24ebe943b84ad4de48bf0514ba83eda_l3.png)
- Der Abstand zwischen zwei Punkten ist die Länge des Verbindungsvektors. Berechne also als erstes den Verbindungsvektor zwischen A und B:
![Rendered by QuickLaTeX.com \[\overrightarrow{AB} = \vec{B} - \vec{A} = \begin{pmatrix}0 \\8 \\1\end{pmatrix}-\begin{pmatrix}4 \\2 \\0\end{pmatrix}=\begin{pmatrix}-4\\6\\1\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-593553108bc845cc8a837b0732fde053_l3.png)
Als nächstes berechnest du die Länge von :
:![Rendered by QuickLaTeX.com \[ |\overrightarrow{AB}| = \left| \begin{pmatrix} -4 \\ 6 \\ 1 \end{pmatrix} \right| = \sqrt{(-4)^2 + 6^2 + 1^2} = \sqrt{16 + 36 + 1} = \sqrt{53}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-77e4048b0f00abddf010a7553dcdf3d0_l3.png)
Also beträgt der Abstand zwischen A und B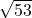 , also ungefähr 7,28.
, also ungefähr 7,28.
-
Du kannst jede Bewegung der Drohne als Vektor darstellen. Lege den Startpunkt als (0|0|0) fest und du kannst die aktuelle Position ausrechnen. Addiere dafür die Bewegungsvektoren deiner Drohne. Im klassischen Koordinatensystem entspricht eine Bewegung nach oben einer Bewegung in x3-Richtung. Nach rechts in x2-Richtung und nach hinten in x1-Richtung. Die aktuelle Position kannst du also mit der folgenden Vektoraddition berechnen:
![Rendered by QuickLaTeX.com \[\begin{pmatrix}0 \\0 \\30\end{pmatrix}+\begin{pmatrix}0 \\10 \\0 \end{pmatrix}+\begin{pmatrix}0 \\0 \\-2\end{pmatrix}+\begin{pmatrix}0 \\-7 \\0 \end{pmatrix}+\begin{pmatrix}-8 \\0 \\0\end{pmatrix}+\begin{pmatrix}0 \\0 \\2\end{pmatrix}=\begin{pmatrix}-8 \\10 - 7 \\30 - 2 + 2\end{pmatrix}=\begin{pmatrix}-8 \\3 \\30\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-cea8f63f83db9d3a924e5621600ebc74_l3.png)
Das ist der Ortsvektor der Drohne. Ihren Abstand zum Startpunkt (0|0|0) erhältst du, indem du die Länge des Ortsvektors berechnest.![Rendered by QuickLaTeX.com \[ \left| \begin{pmatrix} -8 \\ 3 \\ 30 \end{pmatrix} \right| = \sqrt{(-8)^2 +3^2 + 30^2} = \sqrt{64 + 9 + 900} = \sqrt{975} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-decd16488355c410a78c652304c7a0c2_l3.png)
Die Drohne ist also Meter, also ungefähr 31,22 Meter vom Startpunkt entfernt.
Meter, also ungefähr 31,22 Meter vom Startpunkt entfernt.
Vektorrechnung Multiplikation
Du kannst einen Vektor auch mit einer reellen Zahl
r multiplizieren. Du nennst r in diesem Fall ein Skalar. Deshalb heißt die Rechenart auch skalare Multiplikation.
Das Ergebnis erhältst du, indem du jeden Eintrag des Vektors mit r multiplizierst.
![Rendered by QuickLaTeX.com \[\textcolor{red}{r} \cdot \textcolor{blue}{\begin{pmatrix} 2 \\ 0 \\ 5 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{r} \cdot \textcolor{blue}{2} \\ \textcolor{red}{r} \cdot \textcolor{blue}{0} \\ \textcolor{red}{r} \cdot \textcolor{blue}{5} \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-01524d3c8b4e49046d60a3a093b1e270_l3.png)
Geometrisch wird dabei der Vektor um den Faktor r verlängert. Der Vektor  zeigt also in die gleiche Richtung wie
zeigt also in die gleiche Richtung wie  , ist aber doppelt so lang. Der Vektor
, ist aber doppelt so lang. Der Vektor  ist genauso lang wie
ist genauso lang wie  , aber zeigt in die entgegengesetzte Richtung.
, aber zeigt in die entgegengesetzte Richtung.
Vektorrechnung Multiplikation Aufgaben:
- Berechne:
![Rendered by QuickLaTeX.com \[6 \cdot \begin{pmatrix}9 \\8 \\7\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-f41806ec50c192a62197551974735909_l3.png)
- Berechne:
![Rendered by QuickLaTeX.com \[2 \cdot \begin{pmatrix}4 \\6 \\8\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-c8adcf3d7c1751f4ebc16452a8a8db32_l3.png)
- Welcher Vektor hat die gleiche Länge wie
 und zeigt in die entgegengesetzte Richtung?
und zeigt in die entgegengesetzte Richtung?
Vektorrechnung Multiplikation Lösungen:
Skalarprodukt
Das Skalarprodukt ist trotz des ähnlichen Namens etwas anderes als die Skalarmultiplikation.
![Rendered by QuickLaTeX.com \[ \textcolor{red}{\vec{a}} \circ \textcolor{blue}{\vec{b}} = \textcolor{red}{\begin{pmatrix} a_1 \\ a_2 \\ a_3 \end{pmatrix}} \circ \textcolor{blue}{\begin{pmatrix} b_1 \\ b_2 \\ b_3 \end{pmatrix}} = \textcolor{red}{a_1} \cdot \textcolor{blue}{b_1} + \textcolor{red}{a_2} \cdot \textcolor{blue}{b_2} + \textcolor{red}{a_3} \cdot \textcolor{blue}{b_3} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-323e3f0224691c1573c88cf3f4f32a36_l3.png)
Beim Skalarprodukt multiplizierst du den ersten Eintrag von  mit dem ersten Eintrag von
mit dem ersten Eintrag von  , den zweiten Eintrag von
, den zweiten Eintrag von  mit dem zweiten Eintrag von
mit dem zweiten Eintrag von  und den dritten Eintrag von
und den dritten Eintrag von  mit dem dritten Eintrag von
mit dem dritten Eintrag von  . Die Ergebnisse dieser drei Multiplikationen addierst du dann und erhältst das Ergebnis.
. Die Ergebnisse dieser drei Multiplikationen addierst du dann und erhältst das Ergebnis.
Am besten lernst du das an einem konkreten Beispiel:
![Rendered by QuickLaTeX.com \[ \textcolor{red}{\begin{pmatrix} 1 \\ 8 \\ 7 \end{pmatrix}} \circ \textcolor{blue}{\begin{pmatrix} 2 \\ 5 \\ 6 \end{pmatrix}} = \textcolor{red}{1} \cdot \textcolor{blue}{2} + \textcolor{red}{8} \cdot \textcolor{blue}{5} + \textcolor{red}{7} \cdot \textcolor{blue}{6} = 2 + 40 + 42 = 84 \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-60b794bece04caa60861377e97ed75aa_l3.png)
Geometrisch verrät dir das Skalarprodukt, ob zwei Vektoren im rechten Winkel zueinander stehen oder nicht: Zwei Vektoren stehen genau dann im rechten Winkel zueinander, wenn sie das Skalarprodukt 0 haben. Beachte aber, dass dabei keiner der beiden Vektoren der Nullvektor sein darf.
Beispiel:
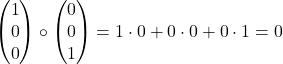
Die beiden Vektoren stehen also im rechten Winkel aufeinander.
Skalarprodukt Aufgaben:
Stehen die beiden Vektoren  und
und  senkrecht aufeinander?
senkrecht aufeinander?
Skalarprodukt Lösungen:
-
![Rendered by QuickLaTeX.com \[\vec{a} \circ \vec{b} =\begin{pmatrix}2 \\2 \\3\end{pmatrix}\circ\begin{pmatrix}5 \\4 \\-6\end{pmatrix}= 2 \cdot 5 + 2 \cdot 4 + 3 \cdot (-6) = 10 + 8 - 18 = 0\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-1ec9ee5824d137e96c7b19f0b71d588e_l3.png)
Da das Skalarprodukt der beiden Vektoren 0 und Vektor der Nullvektor ist, stehen sie im rechten Winkel zueinander. -
![Rendered by QuickLaTeX.com \[\vec{a} \circ \vec{b} =\begin{pmatrix}1 \\2 \\3\end{pmatrix}\circ\begin{pmatrix}4 \\5 \\6\end{pmatrix}= 1 \cdot 4 + 2 \cdot 5 + 3 \cdot 6 = 4 + 10 + 18 = 32\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-ca9a8bab7b8515d9241d855c472d85c9_l3.png)
Da das Skalarprodukt der beiden Vektoren nicht 0 ist, stehen sie nicht im rechten Winkel zueinander.
Kreuzprodukt
Die letzte Art von Vektorrechnungen ist das Kreuzprodukt
, auch oft Vektorprodukt genannt, weil man zwei Vektoren  und
und  multipliziert und einen Vektor als Ergebnis erhält. Dieser Vektor steht dann übrigens immer senkrecht auf
multipliziert und einen Vektor als Ergebnis erhält. Dieser Vektor steht dann übrigens immer senkrecht auf  und
und  .
.
Das Kreuzprodukt berechnest du so:
![Rendered by QuickLaTeX.com \[ \textcolor{red}{\vec{a}} \times \textcolor{blue}{\vec{b}} = \textcolor{red}{\begin{pmatrix} a_1 \\ a_2 \\ a_3 \end{pmatrix}} \times \textcolor{blue}{\begin{pmatrix} b_1 \\ b_2 \\ b_3 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{a_2} \cdot \textcolor{blue}{b_3} - \textcolor{red}{a_3} \cdot \textcolor{blue}{b_2} \\ \textcolor{red}{a_3} \cdot \textcolor{blue}{b_1} - \textcolor{red}{a_1} \cdot \textcolor{blue}{b_3} \\ \textcolor{red}{a_1} \cdot \textcolor{blue}{b_2} - \textcolor{red}{a_2} \cdot \textcolor{blue}{b_1} \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-e0e80a95b17826d163b33d4df794a855_l3.png)
Ein konkretes Beispiel mit Zahlen rechnest du also so aus:
![Rendered by QuickLaTeX.com \[\textcolor{red}{\begin{pmatrix} 1 \\ 8 \\ 7 \end{pmatrix}} \times \textcolor{blue}{\begin{pmatrix} 2 \\ 5 \\ 6 \end{pmatrix}} = \begin{pmatrix} \textcolor{red}{8} \cdot \textcolor{blue}{6} - \textcolor{red}{7} \cdot \textcolor{blue}{5} \\ \textcolor{red}{7} \cdot \textcolor{blue}{2} - \textcolor{red}{1} \cdot \textcolor{blue}{6} \\ \textcolor{red}{1} \cdot \textcolor{blue}{5} - \textcolor{red}{8} \cdot \textcolor{blue}{2} \end{pmatrix} = \begin{pmatrix} 48 - 35 \\ 14 - 6 \\ 5 - 16 \end{pmatrix} = \begin{pmatrix} 13 \\ 8 \\ -11 \end{pmatrix} \]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-1a36578b9a9570adc2601c4ec56d8e4c_l3.png)
Du siehst, dass es eine etwas längere Rechnung ist. Deshalb sind zwei Tipps von uns:
- Schreibe den gesamten Rechenweg auf; so wie er hier steht.
- Übe das Kreuzprodukt, damit du den Ablauf kannst.
Kreuzprodukt Aufgaben:
Finde einen Vektor, der senkrecht auf den beiden Vektoren  und
und  steht mit:
steht mit:
Kreuzprodukt Lösungen:
-
![Rendered by QuickLaTeX.com \[\vec{a} \times \vec{b} =\begin{pmatrix}6 \\5 \\4\end{pmatrix}\times\begin{pmatrix}3 \\2 \\1\end{pmatrix}=\begin{pmatrix}5 \cdot 1 - 4 \cdot 2 \\4 \cdot 3 - 6 \cdot 1 \\6 \cdot 2 - 5 \cdot 3\end{pmatrix}=\begin{pmatrix}-3 \\6 \\-3\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-73a9dbb19f6816f03ce931c6f5c3617f_l3.png)
Das ist also ein senkrechter Vektor zu und
und  . Das reicht als Antwort auf die Aufgabe.
. Das reicht als Antwort auf die Aufgabe.
Falls du aber sichergehen möchtest, dass du dich nicht verrechnet hast, kannst du ganz einfach mit dem Skalarprodukt überprüfen, ob der Vektor auch wirklich senkrecht auf und
und  steht.
steht.![Rendered by QuickLaTeX.com \[\vec{a} \circ\begin{pmatrix}-3 \\6 \\-3\end{pmatrix}=\begin{pmatrix}6 \\5 \\4\end{pmatrix}\circ\begin{pmatrix}-3 \\6 \\-3\end{pmatrix}=6 \cdot (-3) + 5 \cdot 6 + 4 \cdot (-3) = -18 + 30 - 12 = 0\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-cf063f56eadb601052c566437ae08902_l3.png)
![Rendered by QuickLaTeX.com \[\vec{b} \circ\begin{pmatrix}-3 \\6 \\-3\end{pmatrix}=\begin{pmatrix}3 \\2 \\1\end{pmatrix}\circ\begin{pmatrix}-3 \\6 \\-3\end{pmatrix}=3 \cdot (-3) + 2 \cdot 6 + 1 \cdot (-3) = -9 + 12 - 3 = 0\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-21bd10b4f8e72ee2cde07820238beb82_l3.png)
Das Skalarprodukt ist in beiden Fällen 0. Also ist der Vektor auf und auf
und auf  senkrecht. Du hast dich also nicht verrechnet.
senkrecht. Du hast dich also nicht verrechnet. - Hier ist jeder Vektor auf der
 -Achse eine richtige Antwort, weil diese senkrecht auf der
-Achse eine richtige Antwort, weil diese senkrecht auf der  – und der
– und der  -Achse steht. Überprüfe aber am besten zur Übung, ob du mit dem Kreuzprodukt auf dasselbe Ergebnis kommst.
-Achse steht. Überprüfe aber am besten zur Übung, ob du mit dem Kreuzprodukt auf dasselbe Ergebnis kommst.![Rendered by QuickLaTeX.com \[\vec{a} \times \vec{b} =\begin{pmatrix}1 \\0 \\0\end{pmatrix}\times\begin{pmatrix}0 \\1 \\0\end{pmatrix}=\begin{pmatrix}0 \cdot 0 - 0 \cdot 1 \\0 \cdot 0 - 1 \cdot 0 \\1 \cdot 1 - 0 \cdot 0\end{pmatrix}=\begin{pmatrix}0 \\0 \\1\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-a1675db4f0f479878fee81ec356854b0_l3.png)
Das stimmt mit unserer vorherigen Überlegung überein.
Schnittgerade zweier Ebenen
Jetzt beherrschst du sämtliche Operationen der Vektorrechnung. Sehr gut gebrauchen kannst du dieses Wissen, wenn in deiner nächsten Prüfung nach der Schnittgeraden zweier Ebenen gefragt wird. Um darauf vorbereitet zu sein, solltest du dir unbedingt unser Video dazu ansehen.


![Rendered by QuickLaTeX.com \[6 \cdot \begin{pmatrix}9 \\8 \\7\end{pmatrix} = \begin{pmatrix}6 \cdot 9 \\6 \cdot 8 \\6 \cdot 7\end{pmatrix} =\begin{pmatrix}54 \\48 \\42\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-7d9b126ab1d8dcd33f59cefd513e64fa_l3.png)
![Rendered by QuickLaTeX.com \[2 \cdot \begin{pmatrix}4 \\6 \\8\end{pmatrix} = \begin{pmatrix}2 \cdot 4 \\2 \cdot 6 \\2 \cdot 8\end{pmatrix} =\begin{pmatrix}8 \\12 \\16\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-aec195cb6927f838e412d202be34f665_l3.png)
![Rendered by QuickLaTeX.com \[-\vec{a}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-9f97dbf111f26f848f2d67fd9e60cc7f_l3.png)
![Rendered by QuickLaTeX.com \[\vec{a} =\begin{pmatrix}2 \\2 \\3\end{pmatrix}\;\vec{b} = \begin{pmatrix}5 \\4 \\-6\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-b2dbf3aa313a183f4b23631a81591605_l3.png)
![Rendered by QuickLaTeX.com \[\vec{a} = \begin{pmatrix}1 \\2 \\3\end{pmatrix}\;\vec{b} = \begin{pmatrix}4 \\5 \\6\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-ed29080874ebf6969a30c51169a164eb_l3.png)
![Rendered by QuickLaTeX.com \[\vec{a} = \begin{pmatrix}6 \\5 \\4\end{pmatrix}\;\vec{b} = \begin{pmatrix}3 \\2 \\1\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-fbf91e7ae6a7100fc18508c68ff1647b_l3.png)
![Rendered by QuickLaTeX.com \[\vec{a} = \begin{pmatrix}1 \\0 \\0\end{pmatrix}\;\vec{b} = \begin{pmatrix}0 \\1 \\0\end{pmatrix}\]](https://blog.assets.studyflix.de/wp-content/ql-cache/quicklatex.com-0b4e758a6b6200eb2b59bb9f184ef6e5_l3.png)
